Dirac Equation for Energy-Dependent Potential With Energy-dependent Tensor Interaction

Authors

  • C. A. Onate Department of Physics, Kogi State University Anyigba, Nigeria.
  • M. O. Oluwayemi Department of Physical Sciences, Landmark University, Omu- Aran, Nigeria.; Landmark SDG 4 (Quality Education)
  • I. B. Okon Physics Department, University of Uyo, Uyo, Nigeria

Keywords:

Bound state, Dirac equation, Eigen solutions, Wave equation, potential function

Abstract

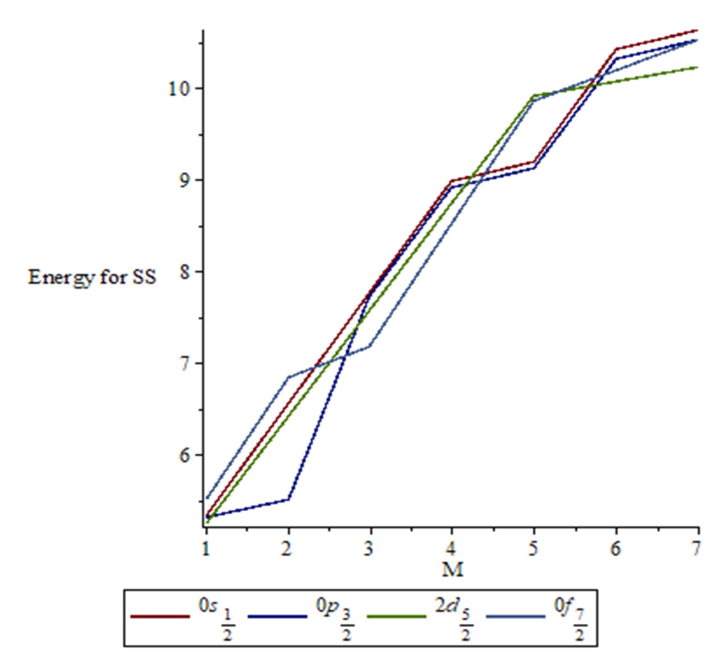
The relativistic symmetries of the Dirac equation were investigated with an energy-dependent tensor potential interaction for two different energy-dependent potentials under parametric Nikiforov-Uvarov method and supersymmetric quantum mechanics and shape-invariance method. It is observed that the energy-dependent tensor interaction has stronger removal effect of the energy degeneracies in both the spin and pseudospin symmetries than the non-energy-dependent tensor interaction.

Dimensions

D. Troltenier, C. Bahri & J.P. Draayer, “Effects of pairing in the pseudo-SU(3) model”, Nucl. Phys. A 586 (1995) 75.

A.E. Stuchbery, “Magnetic properties of rotational states in the pseudo-Nilsson model”, Nucl. Phys. A 700 (2002) 83.

A. Bohr, I. Hamamoto & B.R, “Motteison, Pseudospin in rotating nuclear potentials”, Phys. Scr. 26 (1982) 267.

W. Nazarewicz, P.J. Twin, P. Fallon & J.D. Garrett, “Natural-parity states in superdeformed bands and pseudo SU(3) symmetry at extreme conditions”, Phys. Rev. Lett. 64 (1990) 1654.

A.E. Stuchbery, Magnetic behaviour in the pseudo-Nilsson model,” J. Phys. G. 25 (1999) 611.

D. Troltenier, W. Nazarewicz, Z. Szymanski & J.P. Draayer, “On the validity of the pseudo-spin concept for axially symmetric deformed nuclei”, Nucl. Phys. A 567 (1994) 591.

B.J. Falaye, “Arbitrary `-state solutions of the hyperbolical potential by the asymptotic iteration method”, Few-Body Syst. 53 (2012) 557.

A. Soylu, O. Bayrak & I. Boztosun, “An approximate solution of Dirac-Hulthen problem with pseudospin and spin symmetry for any state”, J. Math. Phys. 48 (2007) 082302

H. Hassanabadi, E. Maghsoodi, S. Zarrinkamar & H. Rahimov, “An approximate solution of the Dirac equation for hyperbolic scalar and vector potentials and a Coulomb tensor interaction by SUSYQM”, Mod. Phys. Lett. A 26 (2011) 2703.

C.A. Onate & J.O. Ojonubah, “Relativistic and nonrelativistic solutions of the generalized Pöschl-Teller and hyperbolical potentials with some thermodynamic properties”, Int. J. Mod. Phys. E 24 (2015) 1550020.

S.M. Ikhdair & R. Sever, “Two approximation schemes to the bound states of the Dirac-Hulthen problem”, J. Phys. A: Math. Theor. 44 (2011) 355301.

M. Hamzavi, A.A. Rajabi & H. Hassanabadi, “Exact Solutions of Dirac Equation with Hartmann Potential by Nikiforov-Uvarov Method”, Int. J. Mod. Phys. A 26 (2010) 1363.

M. C. Onyeaju, A.N. Ikot, C.A. Onate, O. Ebomwonyi, M.E. Udoh & J.O.A. Idiodi, “Approximate bound states solution of the Dirac equation with some thermodynamic properties for the deformed Hylleraas plus deformed Woods-Saxon potential”, Eur. Phys. J. Plus 132 (2017) 302.

J.N. Ginocchio, “The relativistic foundations of pseudospin symmetry in nuclei”, Nucl. Phys. A 654 (1999) 663c.

J.N. Ginocchio¸ “A relativistic symmetry in nuclei”, Phys. Rep. 315 (1999) 231.

G.F. Wei & S.H. Dong, “Algebraic approach to pseudospin symmetry for the Dirac equation with scalar and vector modified Pöschl-Teller potentials”, European Physics Letter (EPL) 87 (2009) 40004.

C. Tezcan & R. Sever, “A general approach for the exact solution of the Schrödinger equation”, Int. J. Theor. Phys. 48 (2009) 337.

I. J. Njoku, C. P. Onyenegecha , C. J. Okereke, A. I. Opara, U. M. Ukewuihe, F. U. Nwaneho, “Approximate solutions of Schrodinger equation and thermodynamic properties with Hua potential”, Results in Physics 24 (2021) 104208.

R. Khordad, B. Mirhosseini, Linear and nonlinear optical properties in spherical quantum dots: Rosen-Morse potential. Opt. Spectroscopy 117 (2014) 440

A. Ghanbari, R. Khordad, & F. Taghizadeh, “Influence of Coulomb term on thermal properties of fluorine”, Chem. Phys. Lett. 801 (2022) 139725.

J.O.A. Idiodi & C.A. Onate, “Entropy, Fisher Information and Variance with Frost-Musulin Potenial”, Commun. Theor. Phys. 66 (2016) 269.

F. Cooper, A. Khare & U. Sukhatme, “Supersymmetry and quantum mechanics”, Phys. Rep. 251 (1995) 267.

F. Cooper, J.N. Ginocchio & A. Wipf, “Derivation of the S-Matrix using super symmetry”, Phys. Lett. A 129 (1988) 145.

F. Cooper, A. Khare & U. Sukhatme, “Relationship between supersymmetry and solvable potentials”, Phys. Rev. D 36 (1987) 2458.

A. Khare & U. Sukhatme, “New shape-invariant potentials in supersymmetric quantum mechanics”, J. Phys. A 26 (1993) L901.

E. Witten, “Dynamical breaking of supersymmetry”, Nucl. Phys. B 185 (1981) 513.

L.E. Gendenshtein, “Derivation of exact spectra of the Schrodinger equation by means of supersymmetry”, Sov. Phys.: JETP Lett. 38 (1983) 356.

C.S. Jia, X.G. wang, X.K. Yao, P.C. Chen & W. Xiao, “A unified recurrence operator method for obtaining normalized explicit wave functions for shape-invariant potentials”, J. Phys A: Math. Gen. 31 (1998) 4763.

C. A. Onate, G. O. Egharevba, & D. T. Bankole, “Eigensolution to Morse potential for Scandium and Nitrogen Monoiodides”, J. Nig. Soc. Phys. Sci. 3 (2021) 286.

Published

2023-01-14

How to Cite

Dirac Equation for Energy-Dependent Potential With Energy-dependent Tensor Interaction. (2023). Journal of the Nigerian Society of Physical Sciences, 5(1), 917. https://doi.org/10.46481/jnsps.2023.917

Issue

Volume 5, Issue 1, February 2023

Section

Original Research
Copyright & Licensing

Copyright (c) 2023 C. A. Onate, M. O. Oluwayemi, I. B. Okon

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

How to Cite

Dirac Equation for Energy-Dependent Potential With Energy-dependent Tensor Interaction. (2023). Journal of the Nigerian Society of Physical Sciences, 5(1), 917. https://doi.org/10.46481/jnsps.2023.917