Stability and Sensitivity Analysis of Dengue-Malaria Co-Infection Model in Endemic Stage

Solomon A. Ayuba; Imam Akeyede; Adeyemi Olagunju

doi:10.46481/jnsps.2021.196

Authors

Solomon A. Ayuba
[email protected]
Federal University of Lafia, P.M.B 146 Lafia, Nasarawa state, Nigeria https://orcid.org/0000-0001-5510-5617
I. Akeyede Federal University of Lafia, P.M.B 146 Lafia, Nasarawa state, Nigeria https://orcid.org/0000-0001-5302-248X
A. S. Olagunju Federal University of Lafia, P.M.B 146 Lafia, Nasarawa state, Nigeria https://orcid.org/0000-0001-6719-5859

Keywords:

Coinfection, Dengue, Malaria, Sensitivity Analysis

Abstract

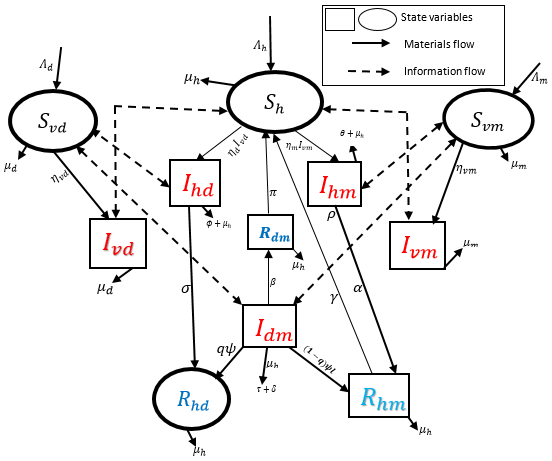
In this study, a deterministic co-infection model of dengue virus and malaria fever is proposed. The disease free equilibrium point (DFEP) and the Basic Reproduction Number is derived using the next generation matrix method. Local and global stability of DFEP is analyzed. The result show that the DFEP is locally stable if R0dm < 1 but may not be asymptotically stable. The value of R0dm calculated is 19.70 greater than unity; this implies that dengue virus and malaria fever are endemic in the region. To identify the dominant parameter for the spread and control of the diseases and their co-infection, sensitivity analysis is investigated. From the numerical simulation, increase in the rate of recovery for co-infected individual contributes greatly in reducing dengue and malaria infections in the region. Decreasing either dengue or malaria contact rate also play a significant role in controlling the co-infection of dengue and malaria in the population. Therefore, the center for disease control and policy makers are expected to set out preventive measures in reducing the spread of both diseases and increase the approach of recovery for the co-infected individuals.

Dimensions

REFERENCES

P. Yongzhen, L. Shgaoying, L. Shuping, & L Changguo, “A delay SEIQR epidemic model with impulse vaccination and the quarantine measure”, Computers and Mathematics with Applications 58 (2009) 135.

W. Viroj, “Concurrent malaria and dengue infection: a brief summary and comment”, Asian Pacific Journal of Tropical Biomedicine, 1 (2011) 326.

World Health Orginization. Fact sheets/detail/malaria report. 2020.

N. F. B. Simo, J. J. Bigna, S. Kenmoe, S. M. Ndangang, E. Temfack, P. F. Moundipa, & M. Demanou, “Dengue virus infection in people residing in africa: a systematic review and meta-analysis of prevalence studies”, Scientific Reports: Nature Research 9 (2019) 3626.

World Health Organization. Dengue cases report. 2019.

J. A. Mensah, K. I. Dontwi, & E. Bonyah, “Stability analysis of multi-infections (malaria, zikka dna elephantiasis)”, Journal of Advances in Mathematics and Computer Science 30 (2018) 2.

M. J. Mutua, F. B. Wang, & K N. Vaidya, “Modeling malaria and typhoid fever co-infection dynamics”, Journal of Mathematical Bioscience 264 (2015) 128.

A. B. Gumel, Z. Mukandavire, W. Garira, & J. M. Tchuenche, “Mathematical analysis of a model for hiv-malaria co-infection”, Mathematical Biosciences and Engineering 6 (2009) 333. doi:10.3934/mbe.2009.6.333

D. Aldila & M. Agustin, “A mathematical model of dengue-chikungunya co-infection in aclosed population”, Journal of physics: Conf. Series 974 (2018) 012001.

E. Bonyah, M. A. Khan, K. O. Okosun, & J. F. G. Aguilar, “On the co-infection of dengue fever and zika virus”, Optimal and Control App Meth 40 (2018) 394.

O. Olawoyin & C. Kribs, “Co-infection, altered vector infectivity, and antibody-dependent enhancement: The dengue-zika interplay”, Society for mathematical biology 83 (2020) 13.

H. T. Alemmeh, “A co-infection model of dengue and leptospirosis diseases”, Advances in Difference Equations 2020 (2020) 664.

T. J. Oluwafemi, E. Azuaba, & Y. M. Kura, “Stablity analysis of disease free equilibrium of malaria, dengue and typhoid triple infection model”, Asian Research Journal of Mathematics, 16 (2020) 11.

T. J. Oluwafemi, N. I. Akinwande, R. O. Olayiwola, & A. F. Akuta, “Co-infection model formulation to evaluate the transmission dynamics of malaria and dengue fever virus”, J. Appl. Sci. Environ. Manage 24 (2020) 7.

O. Gabriel, K. J. Koske, & M. MutisoJohn, “Transmission dynamics and optimal control of malaria in kenya”, Discrete Dynamis in Nature and Society 2016 (2016) 1.

A. Aurelio delos Reyes & J. M. L. Escaner. “Dengue in the Philippines: model and analysis of parameters affecting transmission”, Journal of Biological Dynamics 12 (2018) 894.

M. Derouich & A. Boutayeb, “Dengue fever: Mathematical modelling and computer simulation”, Applied Mathematics and Computation 177 (2006) 528.

S. Syafruddin & N. Lyapunov, “function of sir and seir model for transmission of dengue fever disease”, Int. J. Simulation and Process Modelling, 8 (2013) 177.

U. D. P. Fatmawati & J. Nainggolan, “Parameter estimation and sensitivity analysis of malaria model”, Journal of physics Conference Series 1490 (2020) 012039. DOI: 10.1088/1742- 6596/1490/012039

S. Olaniyi & O. S. Obabiyi, “Mathematical model for malaria transmission dynamics in human and mosquito populations with nonlinear forces of infection”, International Journal of Pure and Applied Mathematics 88 (2013) 125.

Y. Xing, Z. Guo, & J. Liu, “Backward bifurcation in malaria transmission model”, Journal of Biological Dynamics 1 (2020) 14.

H. Yin, C. Yang, & X. Zhang, “Dynamics of malaria transmission model with sterile mosquitoes”, J. Bio. Dny. 12 (2018) 577.

C. C. Chavez, Z. Feng, & W. Huang, “On the computation of r and its role on global stability”, //www.researchgate.net/profile/Carlos Castillochavez2/publication/228915276, Biometric Unit Technical Report M1553 (2001) 1.